Acceleration Due to Gravity
Acceleration Due to Gravity: Overview
This Topic covers sub-topics such as Acceleration Due to Gravity, Binomial Expansion, Binomial Approximation, Acceleration Due to Gravity in Terms of Density and Radius of Planet and, Graph of Acceleration Due to Gravity vs Radial Distance
Important Questions on Acceleration Due to Gravity
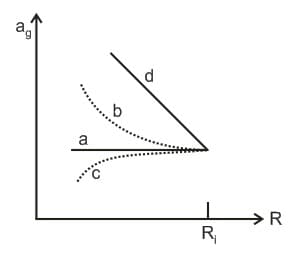
A (nonrotating) star collapses onto itself from an initial radius with its mass remaining unchanged. Which curve in figure best gives the gravitational acceleration on the surface of the star as a function of the radius of the star during the collapse?
If a tunnel is cut at any orientation through the earth, then a ball released from one end will reach the other end in how much time (neglect earth rotation)?
A spherical uniform planet is rotating about its axis. The velocity of a point on its equator is . Due to the rotation of the planet about its axis the acceleration due to gravity at equator is of at poles. The escape velocity of a particle on the planet in terms of from the pole of the planet is,
The mass and diameter of a planet are twice those of earth. What will be the period of oscillation of a pendulum on this planet if it is a second pendulum on earth?
If the radius of the earth be increased by a factor of 5, by what factor its density be changed to keep the value of g same?
Let be the angular velocity of the earth's rotation about its axis. Assume that the acceleration due to gravity on the earth's surface has the same value at the equator and the poles. An object weighed at the equator gives the same reading as a reading taken at a depth below earth's surface at a pole . The value of is:
A man is standing on an international space station, which is orbiting earth at an altitude with a constant speed . If the man's weight is , his acceleration is
A mass is attached to a spring that hangs vertically. The extension produced in the spring is on Earth. The acceleration due to gravity on the surface of the Moon is one-sixth of its value on the surface of the Earth. The extension of the spring on the Moon would be :
Which one of the following statements is true?
Two planets A and B of radii and have densities and respectively. The ratio of acceleration due to gravity at the surface of B to A is:
The radii of two planets and are and and their densities are and respectively. The ratio of acceleration due to gravity at their surfaces will be
The weight of a body on the earth is Then weight of the body when taken to a depth half of the radius of the earth will be:
Assuming the earth to be a sphere of uniform mass density, the weight of a body at a depth from the surface of earth, if its weight on the surface of earth is , will be : (Given radius of earth)
The weight of a body on the surface of the earth is . The gravitational force on it when taken at a height, from the surface of earth, equal to one-fourth the radius of the earth is:
The acceleration due to gravity at height above the earth if (Radius of earth) is given by
Given below are two statements:
Statement I : Rotation of the earth shows effect on the value of acceleration due to gravity .
Statement II : The effect of rotation of the earth on the value of at the equator is minimum and that at the pole is maximum.
In the light of the above statements, choose the correct answer from the options given below
An object weighs at the surface of Earth. Find the weight at a depth of , where is the radius of Earth.
If the weight of an object on earth's surface is , then weight of the same particle at a depth from surface would be ( is radius of earth)
If is the weight on the surface of Earth then weight of same body at a height above the surface of Earth is equal to
( Radius of Earth)
Assuming that the earth is a sphere of radius with uniform density, the distance from its centre at which the acceleration due to gravity is equal to ( is the acceleration due to gravity on the surface of earth) is
